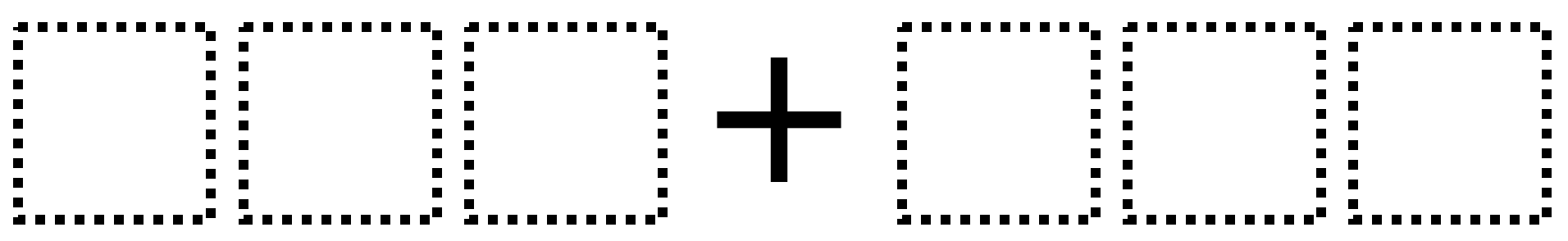
Directions: Arrange the digits 1-6 into two 3-digit whole numbers. Make the sum as close to 1000 as possible.
Hint
Which digits will be the most helpful to focus on to make the solution as close to 1,000 as possible?
Answer
993 (multiple ways to create the solution)
Source: Ian Kerr
 Open Middle®
Open Middle®



Not able to pick this up in a PDF. Is there a trick to it?
Still not working as a PDF!
Can’t get it to work either……
Last week when I was online at the site, it allowed me to save to google drive as a pdf. Where did that option go? Please help!
Hi Sondra. I just added it back. It was buggy and I thought no one used it. Glad to be wrong and sorry for the inconvenience.
500+500=1000
dc, you can’t use any zeros.
400+600=1000
devn smith, you can’t use any zeros.
Nico 500+500=1000
Daniel J. Lombardo, you can’t use any zeros.
500+500
1.666+334
2.555+445
Two ways to create 1,000 using no zeros
ashton nguyen, you can’t have any zeros.
900+100=1000
444 + 556 = 1000.
I believe you’re supposed to use *all* the digits 1 – 6 in forming the two numbers. (And, you can only use each digit one time…)
500+500
I’m posting a “general” comment, b/c so many responses/comments are erroneously doing one of the following:
1) Including decimal values (problem states that the two numbers must be *whole* numbers)
2) Including the digit zero (not allowed)
3) Failing to use each of the digits 1 – 6 one time each.
500+500=1000
441+559=1000
IM Jr but my name is really YAEL SANTOS
500+500
700+300
399+501
500+500=1,000
900+100=1000
400+600=1,000
you cant have zeros, Sai.M
666+334=1,000
one hundred plus nine hundred equals one thousand
it is so easy.
The answer given above states that the closest sum to 1000 that you can form from the digits 1 – 6 is 993.
I believe there are 16 different ways to do so, with one example being: 431 + 562.
561 + 432 = 993
So close! It is not possible to get to 1,000.