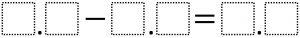
Directions: Using the digits 1-9, subtract two numbers to get a difference closest to 0.
Hint
What is your plan for the digits? Where should you place them? What does it mean for a decimal to be close to 0?
Answer
There could be multiple answers, but we have only found one so far. It is 21 – 19.8 = 1.2
Source: Owen Kaplinsky
 Open Middle®
Open Middle®



Why is the difference (answer) shifted to the right so that the decimal points are misaligned? Are the instructions to use digits more than once? Or can you use the digits only one time? The answer shows multiple up to 3 digits being used, yet the example leads people to use only two digit numbers at each level. Finally, the answer shows 21 as the minuend, yet the example falsely makes me think that I should be using a decimal, like 2.1. Thank you.
Sorry, we’ve fixed this image.
The boxes above are misleading. Your answer: 21 – 19.8 = 1.2 uses 5 digits in your problem, however you only used a model with 4 boxes. Confusing for students. Please add the extra boxes in your problem.
Sorry, we’ve fixed this image.
Sorry…. I don’t see how this image was corrected. It looks like the same problem.
4. 12 – 3.98 is what I found.
Nice! That’s what I got too!
Nice! That’s what I got too!
I think the image is not showing on the page. Like the problem though!
I don’t understand. Why not 2-1.987654321?
Or, 2-1.98765431 I guess.
While the written directions don’t mention this, you are usually meant to put in exactly one digit into each of the boxes in the image. So your decimal numbers each have to have exactly one digit after the decimal point.
8.5-7.3=1.2
Izi
The answer given has the digit 2 repeated, which – while not explicitly against the rules as they are stated here – is contrary to most of the other problems on this website, where each digit can only be used at most once. But it also has 7 digits, when the image only has 5 boxes to put a digit into.
If we can use repeated digits and use as many digits as we want (because the directions don’t mention only one digit going into each box), the closest difference to 0 – like Deborah said – would be 2-1.98765431 = 0.01234569
If we can only fill each box in the image with exactly one digit and repeating digits is allowed, then:
The closest difference to 0 is 1.1, which is the result of 64 different solutions:
2.2 – 1.1 = 1.1
2.3 – 1.2 = 1.1
2.4 – 1.3 = 1.1
2.5 – 1.4 = 1.1
2.6 – 1.5 = 1.1
2.7 – 1.6 = 1.1
2.8 – 1.7 = 1.1
2.9 – 1.8 = 1.1
3.2 – 2.1 = 1.1
3.3 – 2.2 = 1.1
3.4 – 2.3 = 1.1
3.5 – 2.4 = 1.1
3.6 – 2.5 = 1.1
3.7 – 2.6 = 1.1
3.8 – 2.7 = 1.1
3.9 – 2.8 = 1.1
4.2 – 3.1 = 1.1
4.3 – 3.2 = 1.1
4.4 – 3.3 = 1.1
4.5 – 3.4 = 1.1
4.6 – 3.5 = 1.1
4.7 – 3.6 = 1.1
4.8 – 3.7 = 1.1
4.9 – 3.8 = 1.1
5.2 – 4.1 = 1.1
5.3 – 4.2 = 1.1
5.4 – 4.3 = 1.1
5.5 – 4.4 = 1.1
5.6 – 4.5 = 1.1
5.7 – 4.6 = 1.1
5.8 – 4.7 = 1.1
5.9 – 4.8 = 1.1
6.2 – 5.1 = 1.1
6.3 – 5.2 = 1.1
6.4 – 5.3 = 1.1
6.5 – 5.4 = 1.1
6.6 – 5.5 = 1.1
6.7 – 5.6 = 1.1
6.8 – 5.7 = 1.1
6.9 – 5.8 = 1.1
7.2 – 6.1 = 1.1
7.3 – 6.2 = 1.1
7.4 – 6.3 = 1.1
7.5 – 6.4 = 1.1
7.6 – 6.5 = 1.1
7.7 – 6.6 = 1.1
7.8 – 6.7 = 1.1
7.9 – 6.8 = 1.1
8.2 – 7.1 = 1.1
8.3 – 7.2 = 1.1
8.4 – 7.3 = 1.1
8.5 – 7.4 = 1.1
8.6 – 7.5 = 1.1
8.7 – 7.6 = 1.1
8.8 – 7.7 = 1.1
8.9 – 7.8 = 1.1
9.2 – 8.1 = 1.1
9.3 – 8.2 = 1.1
9.4 – 8.3 = 1.1
9.5 – 8.4 = 1.1
9.6 – 8.5 = 1.1
9.7 – 8.6 = 1.1
9.8 – 8.7 = 1.1
9.9 – 8.8 = 1.1
The greatest difference btw is 9.9 – 1.1 = 8.8
If we additionally cannot repeat any digit:
The closest possible difference to 0 is indeed 1.2, as a result of the following 12 of the 400 true statements that don’t repeat a digit:
4.7 – 3.5 = 1.2
4.8 – 3.6 = 1.2
4.9 – 3.7 = 1.2
5.8 – 4.6 = 1.2
5.9 – 4.7 = 1.2
6.9 – 5.7 = 1.2
7.5 – 6.3 = 1.2
8.5 – 7.3 = 1.2
8.6 – 7.4 = 1.2
9.5 – 8.3 = 1.2
9.6 – 8.4 = 1.2
9.7 – 8.5 = 1.2
The one furthest away from 0 is btw 9.7 – 1.2 = 8.5
If we look at the solution suggested by the author (21 – 19.8 = 1.2), we could also assume the task is meant to be the following:
“Fill each blank in _ _ – _ _ . _ = _ . _ with one digit such that the equation holds and the result on both sides of the equation is as small as possible”.
In that case:
There are 71 solutions (with repeated digits allowed) that are equal to 1.1, which is the smallest possible result. Example: 13.9 – 11 = 1.1
The greatest result is 9.9, for example in 99 – 89.1 = 9.9
Quick correction: If we *cannot* use repeated digits, but use as many digits as we want (because the directions don’t mention only one digit going into each box), the closest difference to 0 (without using 0 anywhere, since the digit 0 is not allowed to be used as per the directions) would still be 1.2, because anything below either contains a zero or has the 1 repeated.
If we *can* repeat digits, the closest difference to 0 would be 1.1, because anything below would contain a 0. One example of a solution would then be 9.9 – 8.8 = 1.1 (or in the form of the solution suggested by the author: 21 – 19.9 = 1.1)
3.0-2.9=0.1