Open Middle®
Directions: Using the digits 1 to 9, at most one time each, place a digit in each box to create a quadratic equation such that the distance between the solutions is greater than 1.
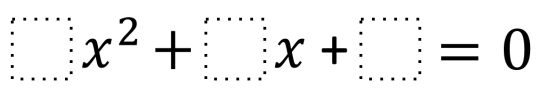
Hint
How does the structure of the quadratic formula help us understand the relationship between the two solutions?
If you are thinking of the problem visually, what effect do the leading coefficient and constant term have on the x-intercepts given that their values are restricted to 1 to 9?
Extensions: what pair of solutions would yield the greatest distance between them? How close to 1 can you make the distance?
Answer
There are many answers including 1x^2+9x+2=0. Other options include any quadratic where a = 1, b is either 4, 5, 6, 7, 8, or 9, and c is less than 8
Source: Mong Kon Mo