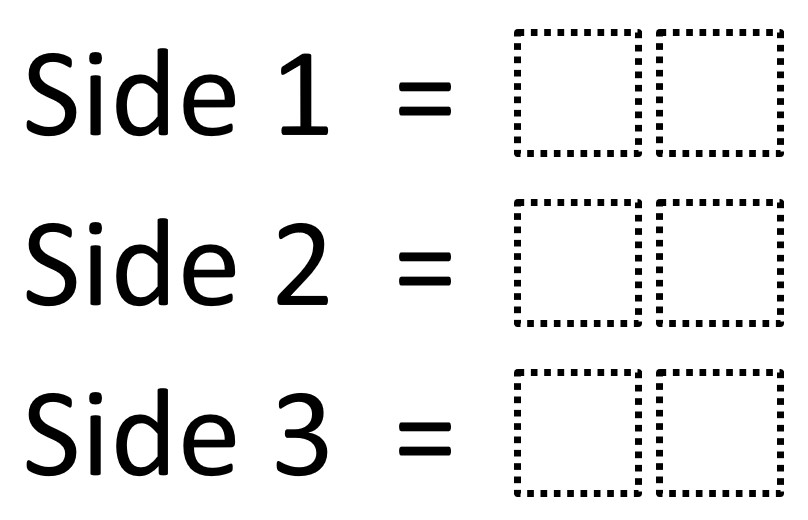
Directions: Using the digits 1 through 6 at most one time each, place a digit in each box to find three side lengths that are two-digits each and form an acute triangle.
Hint
How can you make sure that it is a triangle?
How can you use the Pythagorean Theorem to make sure it is an obtuse triangle?
How is the Pythagorean Theorem for an obtuse triangle than the Pythagorean Theorem for a right triangle?
How can you use the Pythagorean Theorem to make sure it is an obtuse triangle?
How is the Pythagorean Theorem for an obtuse triangle than the Pythagorean Theorem for a right triangle?
Answer
Number of Unique Solutions: 16
1: 23,46,51
2: 25,36,41
3: 26,35,41
4: 31,46,52
5: 31,54,62
6: 32,46,51
7: 32,54,61
8: 36,41,52
9: 36,42,51
10: 41,52,63
11: 42,51,63
12: 42,53,61
13: 43,51,62
14: 52,34,61
15: 52,43,61
16: 53,41,62
1: 23,46,51
2: 25,36,41
3: 26,35,41
4: 31,46,52
5: 31,54,62
6: 32,46,51
7: 32,54,61
8: 36,41,52
9: 36,42,51
10: 41,52,63
11: 42,51,63
12: 42,53,61
13: 43,51,62
14: 52,34,61
15: 52,43,61
16: 53,41,62
Source: Samantha Cruz
 Open Middle®
Open Middle®



The hints reference obtuse triangles rather than acute ones, and the last one seems to be missing a word.
Sorry, it took so long but we believe we finally got this problem updated and corrected.
Is there a way to find the triple without guess and check?
I think there are multiple right answers.