Directions: Using the integers -9 to 9 at most one time each, fill in the boxes to make an equation where the product’s exponent has the greatest possible value.
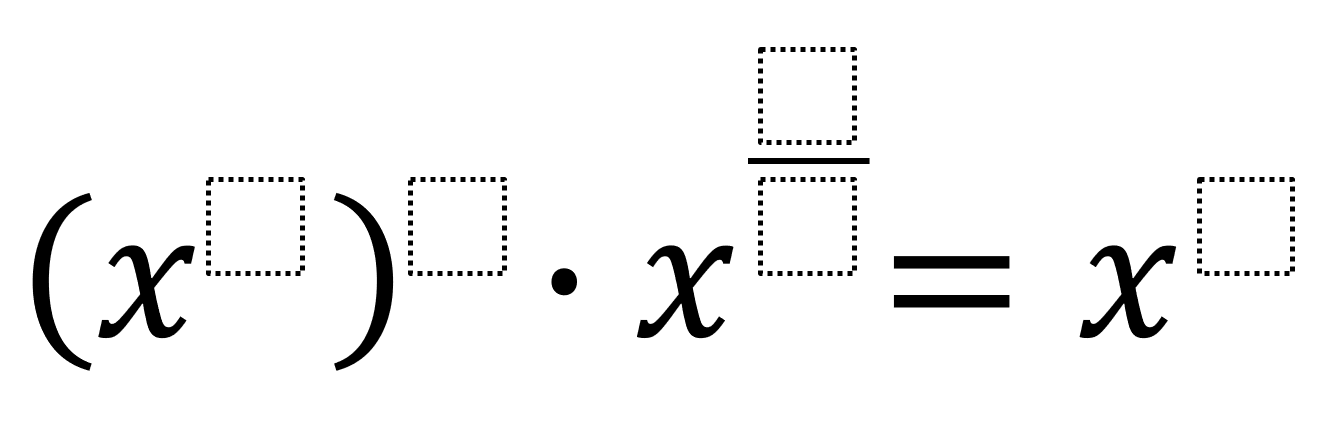
Hint
How do you know when to multiply, divide, subtract, or add the exponents’ value?
Answer
There are many possible answers that make the product’s exponent equal to 9 including:
(x^3)^5 · x^(-6/1) = x^9
(x^3)^5 · x^(-6/1) = x^9
Thanks to everyone in the comments who pointed out that my original answer was wrong.
Source: Robert Kaplinsky
 Open Middle®
Open Middle®



x^15 . x^(1/6) is not equal to x^9 you have to add the exponent which leaves you with an exponent of 15 1/6
I agree Heidi. If the original problem said using from -9 to 9, then a person can use -6/1 rather than 1/6 (which are not the same). This would result in an answer of x^9, assuming everything else remained the same respectively.
If you keep it from the digits 1 to 9 a maximum of once each, you could say (x^5)^1 * x^(8/2) = x^9 or (x^1)^5 * x^(8/2) = x^9. There are some other possibilities that will result in x^9. I can think of 4 more that will also result in x^9 with the original restrictions, so a great way to increase the rigor, develop conceptual understanding, and assess numeracy skills would be to identify as many solutions as possible that result in a product with the greatest exponent.
LOL, I got my own problem wrong for the same misconception I was trying to find out if kids had. Thanks everyone. I will fix it now.
The answer key is definitely wrong!
My tutoree student found a solution to end up with 9 though!
(x^1)^5 x^(8/2)= x^9
LOL, I got my own problem wrong for the same misconception I was trying to find out if kids had. Thanks everyone. I will fix it now.