Directions: Using the digits 1-6, at most one time each, fill in the boxes to create the largest and smallest combined perimeter/circumference for the rectangle and circle.
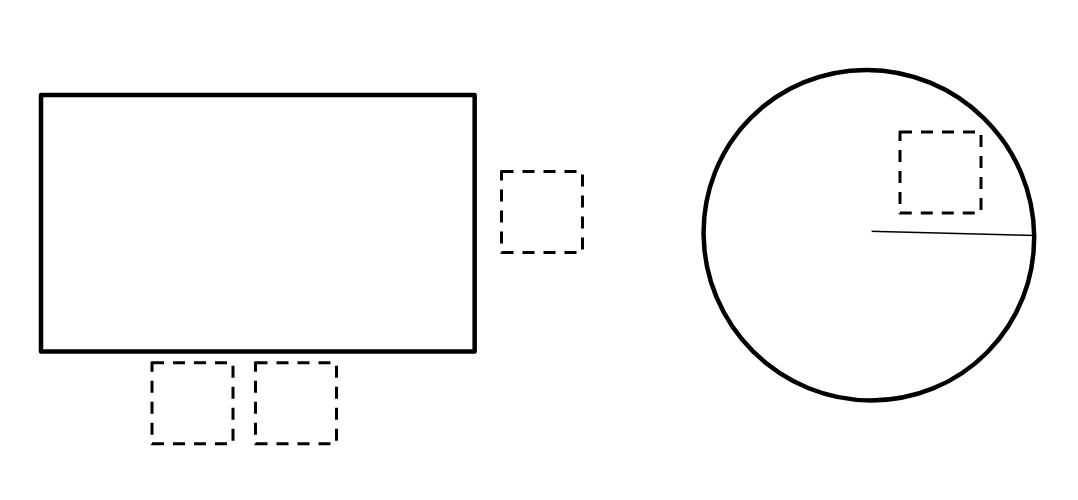
Hint
Which box number change has the greatest effect on the combined perimeter/circumference? Why?
What part of your calculations will be the same every time?
What part of your calculations will be the same every time?
How many permutations are there of the numbers 1-6 in four positions?
Which permutations matter for the largest sum? Why?
Which permutations matter for the smallest sum? Why?
Answer
greatest: (53 · 6) + (2π · 4) = 343.13… units
least: (24 · 1) + (2π · 3) = 42.85… units
Source: Christin Smith
 Open Middle®
Open Middle®



The solution given is actually for area of the rectangle and circumference of the circle
Where do you put the numbers? Like which boxes? Thanks.
You can choose to use any of the digits 1-6 in each of the boxes so that the rectangle has side lengths and the circle has a radius. You can only use each digit once.
David, you are right. With the solution (with given numbers) should look like:
greatest: (2*53+2*6)+(2π · 4)=143.13….
least: (2*24+2*1)+(2π · 3)=68.84…..
HOWEVER: Neither are the greatest or smalles that we found.
Greatest: (2*65+2*4)+(2π · 3)=156.84…
Least: (2*13+2*4)+(2π · 2)=46.56…
Brianna… I believe this is the greatest that we have found
(2*65+2*3) + (2pi * 4) = 161.13
Greatest: Rectangle with sides 63 and 4 and circle with radius 5. Answer: 165.4
This is a solution but the one given in the answers is greater.
It is, but the solution used for the rectangle is the area, not the perimeter.
Least: Rectangle with sides 13 and 4 and circle with radius 2. Answer: 46.56
Similarly with this solution Eric – it works but is not the smallest possible area.
Are we finding the smallest perimeter or area???
My students came up with the following for greatest:
2(64+3) + (5*2*pi) = 134 + 31.4 = 165.4
Us, too. Was super confused by the 313 answer until I really looked at.
I agree with 165.4 as the greatest total.
Note that you can also get that same total by reversing the 3 and the 4:
2(63+4) + (5 * 2*pi)
I wrote a program that solves these. The largest combined perimeter/circumference is the one that was already found with:
A 64 x 3 rectangle and a circle of radius 5 = 165.42
A 63 x 4 rectangle and a circle of radius 5 = 165.42
Least:
A 14 x 3 rectangle and a circle of radius 2 = 46.57
A 13 x 4 rectangle and a circle of radius 2 = 46.57
For combined areas:
Greatest:
A 53 x 6 rectangle and a circle of radius 4 = 368.27
Least:
A 34 x 1 rectangle and a circle of radius 2 = 46.57
This is totally wrong. we were doing this in class and my students came here to find the answer. My students and I found out that they were doing Area and not Perimeter. The biggest one is actually 156.84 and Smallest is actually 46.56.
My class also found that the largest is 165.4:
Perimeter = 2*64 + 2*3 = 134
Circumference = 3.14*2*5 = 31.4
Total = 165.4