Open Middle®
Directions: Using the integers -9 to 9 at most one time each, fill in the boxes twice: once to make a positive real number product and once to make a negative real number product. You may reuse all the integers for each product.
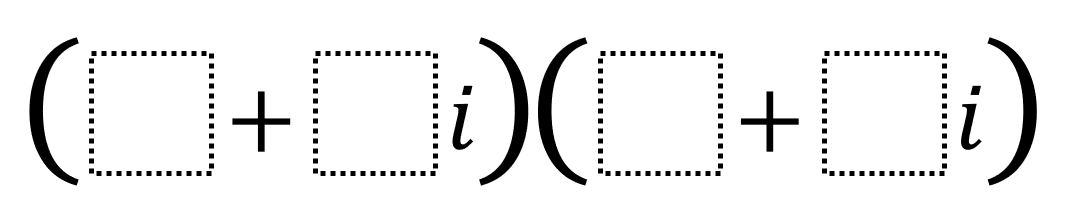
Hint
What is the difference between a real and complex number? What causes an i to go away during multiplication? What causes a term to go away during binomial multiplication? How do we choose integers such that the i terms are additive inverses of one another? Once we find one real number product, how might we find one of the opposite sign?
Answer
There are many answers, but one is (3 + 6i)(2 + -4i) for the positive solution and (-3 + -6i)(2 + -4i) for the negative solution.
Source: Robert Kaplinsky in Open Middle Math
