Open Middle®
Directions: Using the digits 1 to 9 at most one time each, fill in the boxes to create a quadratic equation with the greatest possible maximum value.
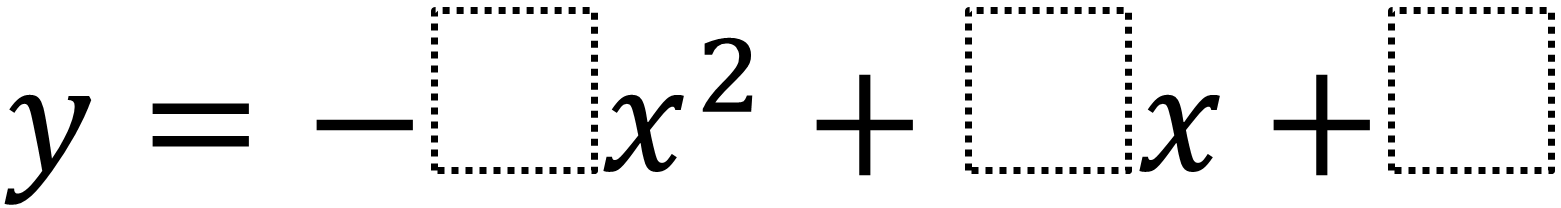
Hint
Where is the best place to put the 9? How does each box affect the graph’s behavior?
Answer
The quadratic with the greatest possible maximum value is -x^2 + 9x + 8. Play with the sliders on this Desmos graph for a hands on way of understanding how the a, b, and c terms affect the quadratic: https://www.desmos.com/calculator/kdsj3ic0gn
Source: Robert Kaplinsky
If x^2-d^2 =0 is known to your student to have solution plus or minus d for solution and horizontal slide is known to your student then when your student slides the quadratic so axis of symmetry is “new” y axis will your student be able to find zeroes real or non real?
Specific f(x)=x^2-2x-3 slide to f(x+1)=x^2-4. Is this not “easy” to solve. Will your student know how to move (-2,0) back to original x cross y?
Can your student find and prove the quadratic formula from this connection of ideas?