Open Middle®
Directions: Using the digits 1 to 9, at most one time each, create 3 different expressions such that their graphs contains any 2 of the 3 following criteria:
1) Horizontal Asymptote @ y = some positive rational number
2) Slant Asymptote with a slope such that: 1 < m ≤ 2
3) Two Vertical Asymptotes
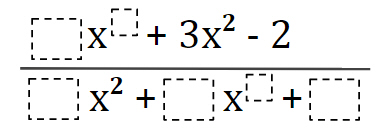
Hint
Is it possible to get a positive and negative asymptote with our restrictions?
Vert Asymptote: First term exponent < 2; Set bottom = Zero Slant Asymptote: First term exponent > 2; Slope set by coefficients of first terms simplified
Horiz. Asymptote: First term exponent = 2: Location by coefficients of first terms simplified
All the above hold true because LARGEST term exponent on bottom is “”2″”
Answer
To get criteria 2 and 3 the top (first term) coefficient must be larger than the bottom
(first term) coefficient… therefore top lead coefficient > 3 in this solution example.
Source: Gregory L. Taylor, Ed.D.
