Directions: In triangle ABC, angle ABC is obtuse. Using the digits 1 to 9 at most one time each, place a digit in each box to make angle ACB the smallest possible acute angle.
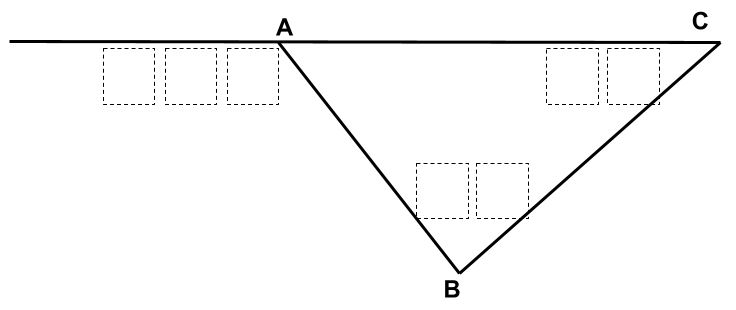
Hint
What does the first number in the three-digit number have to be?
What does the first number in the obtuse angle have to be?
What numbers do not work as the first digit of the acute angle? Why?
What does the first number in the obtuse angle have to be?
What numbers do not work as the first digit of the acute angle? Why?
Answer
42 Degrees
138 = 96 + 42
137 = 95 + 42
138 = 96 + 42
137 = 95 + 42
Source: Jay Sydow
 Open Middle®
Open Middle®



A great extension of this problem would be to remove the restriction that angle ABC is obtuse and additionally pose slightly different questions:
What measures of angle ACB are possible?
What measure(s) of angle ACB yield multiple possible values of angle ABC?
Today, one of my students beat this. She found 37 degrees = angle ACB. The two remote interior angles would be 37 degrees & 89 degrees and would add to the exterior angle of 126 degrees.
89 Degrees is not obtuse as required by the instructions
I had a few kids that found both possibilities pretty early and had them do an extension where they were able to use digits 0-9 instead of 1-9. We determined that 42 was still the best possible answer due to repeating digits.
I extended this to include the numbers 0-9 with my students and they found 4, 98, and 102 as the solution.