Open Middle®
Directions: Using the digits 1 to 9 at most one time each, fill in the boxes to make the equality true:
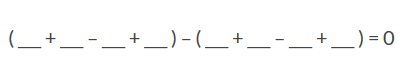
Hint
What numbers will produce a difference of zero?
Answer
Because of the commutative property, there are many different answer combinations. One possible solution would be: ( 9 + 6 – 5 + 2 ) – ( 8 + 7 – 4 + 1 )
Source: Bryan Anderson
Also, (9+1-8+2) – (7+3-6+4) = 0.
that would = -4
you can use those numbers just move them around.
(9+1-8+2)-(7+3-6=4=0
bomba clatt
( 9 + 6 – 5 + 2 ) – ( 8 + 7 – 4 + 1 )
(9+8-4+3)-(7+6-2+5)=0
(9 + 2 – 5 + 6) – (5 + 6 – 2 + 3) = 0
(9+6-5+2)-(8+7-4+1)=0
( 9 + 6 – 5 + 2 ) – ( 8 + 7 – 4 + 1 )
( 5 + 2 – 9 + 6 ) – ( 4 + 1 – 8 + 7 )?????
(8+9-4+3)-(6+7-2+5)=0
Also (4+3-2+8)-(3+4-3+9)=0
Because of the commutative property, there are many different answer combinations. One possible solution would be: ( 9 + 6 – 5 + 2 ) – ( 8 + 7 – 4 + 1 )
(9+6-5+2)-(8+7-4+1)
(9+5-6+2)-(7+8-4+1)
( 9 + 6 – 5 + 2 ) – ( 8 + 7 – 4 + 1 )
( 9 + 6 – 5 + 2 ) – ( 8 + 7 – 4 + 1 )=0
(9+1-3+3)-(5+6-10+9)=0
this one is wrong
no this is 0
However, you cannot use 10. 1-9 only once.
(7+8-2+3)-(6+9-1+4)=0
( 9 + 6 – 5 + 2 ) – ( 8 + 7 – 4 + 1 )
(9+18-6+3)-(7+24-6+7)=0
(9+6-5+2)-(8+7-4+1)
(5+13-3+7)-(7+12-6+3)
(4+5-6+3)-(7+1-6+2)=0
(9+6-5+2)-(8+7-4+1)
(9+6-5+2)-(8+7-4+1)
(2+(-5)+6+9)-(1+(-4)+7+8)
( 1 + 9 – 8 + 2 ) – ( 7 + 3 – 4 + 6 )
(8+2-4+6)-(9+1-7+3)
( 1 + 9 – 8 – 2 ) – ( 7 + 3 – 4 – 6 )
(9+2-1+3)-(4+8-5+6)