Open Middle®
Directions: Using the digits 0 to 9 at most one time each, fill in the boxes to create an inequality whose solution set is x < -1/2.
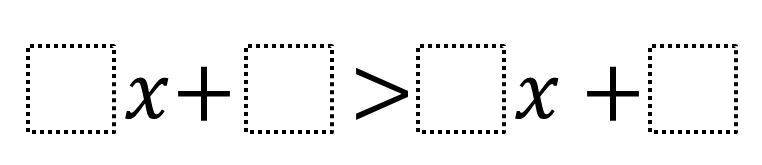
Hint
What does it mean to have a solution set in an inequality? How can you use the solution set “border number” to help you write an inequality?
Answer
There are many correct answers. Some possible responses could be:
2x + 5 > 4x + 6;
1x + 5 > 7x + 8;
9x + 6 < 5x + 4
2x + 5 > 4x + 6;
1x + 5 > 7x + 8;
9x + 6 < 5x + 4
Source: Daniel Luevanos
I love this question. I’ve been wondering what to call the “border number.” I’ve been calling it a “limit.” What are your thoughts on that?
Glad you like the question, Lane! I usually refer to the “border number” or “limit” as the “boundary point.” I never thought about using “limit” as part of my discussion with students, but now I’m going to. Thanks!
Might I suggest “critical point.”
I call them boundaries. That term is very appropriate for both number line inequalities or inequalities with any polynomial function.
My students also came up with these solutions:
1x + 4 > 3x + 5
1x + 5 > 3x + 6
1x + 7 > 3x + 8
1x + 8 > 3x + 9
5x + 8 > 7x + 9
2x + 5 > 4x + 6
4x + 8 > 6x + 9
These are all great solutions! Thank you for sharing!
Oh wow
your students are bright !!!!!!!!!!!!!!!!!. Thanks for building the children of the nation
Thank you very much for the help to develop us students by helping us some difficult questions we can´t manage. May God bless you
Thank you very much for the problem!
I have a student that developed an equation to represent the situation as long as no number is repeated, where x is any number between 1 and 9.
x + (constant) > x +1 + (constant + 2)